decaylet美人双胞胎姐妹
let美人双胞胎姐妹 时间:2021-01-15 阅读:()
AnIntroductiontoTheTwinPrimeConjectureAllisonBerkeDecember12,2006AbstractTwinprimesareprimesoftheform(p,p+2).
Therearemanyproofsfortheinnitudeofprimenumbers,butitisverydiculttoprovewhetherthereareaninnitenumberofpairsoftwinprimes.
Mostmathematiciansagreethattheevidencepointstowardthisconclusion,butnumerousattemptsataproofhavebeenfalsiedbysubsequentreview.
Theproblemitself,oneofthemostfamousopenproblemsinmathematics,hasyieldedanumberofrelatedresults,includingBrun'sconjecture,Mertens'theorems,andtheHardy-LittlewoodConjecture.
Alongwiththeseconjectures,thereareanumberofresultswhichareeasiertoarriveat,butneverthelesshelpmathematiciansthinkabouttheinnitudeofprimes,andthespecialpropertiesoftwinprimes.
Thispaperwillintroducetheaforementionedconjecturesassociatedwiththetwinprimeconjecture,andworkthroughsomeexercisesthatilluminatethedicultiesandintricaciesofthetwinprimeconjecture.
1Introduction:TheOriginalConjectureandFailedProofsThetermtwinprimewascoinedbyPaulStackelinthelatenineteenthcentury.
Sincethattime,mathematicianshavebeeninterestedinthepropertiesofrelatedprimes,bothinrelationtonumbertheoryasawhole,andasspecic,well-denedproblems.
Oneoftherstresultsoflookingattwinprimeswasthediscoverythat,asidefrom(3,5),alltwinprimesareoftheform6n±1.
Thiscomesfromnoticingthatanyprimegreaterthan3mustbeoftheform6n±1.
Toshowthis,notethatanyintegercanbewrittenas6x+y,wherexisanyinteger,andyis0,1,2,3,4or5.
Nowconsidereachyvalueindividually.
Wheny=0,6x+y=6xandisdivisibleby6.
Wheny=1therearenoimmediatelyrecognizablefactors,sothisisacandidateforprimacy.
Wheny=2,6x+2=2(3x+1),andsoisnotprime.
Forthecasewhen·y=3:6x+3=3(2x+1)andisnotprime.
Wheny=4:6x+4=2(3x+2)··andisnotprime.
Wheny=5,6x+5hasnoimmediatelyrecognizablefactors,andisthesecondcandidateforprimacy.
Thenallprimescanberepresentedaseither6n+1or6n1,andtwinprimes,sincetheyareseparatedbytwo,willhavetobe6n1and6n+1.
1TwinPrimeConjecture2Furtherresearchintotheconjecturehasbeenconcernedwithndingexpressionsforaformoftheprimecountingfunctionπ(x)thatdependonthetwinprimeconstant.
Theprimecountingfunctionisdenedasπ(x)={N(p)|px}whereN(p)denotesthenumberofprimes,p.
Onemotivationfordeningtheprimecountingfunctionisthatitcanbeusedtodetermineaformulaforthesizeoftheintervalsbetweenprimes,aswellasgivingusanindicationoftherateofdecaybywhichprimesthinoutinhighernumbers.
Ithasbeenshownalgebraicallythattheprimecountingfunctionincreasesasymptoticallywiththelogarithmicintegral[12].
Inthefollowingexpression,π2(x)referstothenumberofprimesoftheformpandp+2greaterthanx,andisthetwin2primeconstant,whichisdenedbytheexpression(19p11)2)overprimesp2.
ThetermO(x),meaning"ontheorderofx,"isdenedasfollows:iff(x)andg(x)aretwofunctionsdenedonthesameset,thenf(x)isO(g(x))asxgoestoinnityifandonlyifthereexistssomex0andsomeMsuchthat|f(x)|M|g(x)|forxgreaterthanx0.
Thisexpressionforthetwinprimecountingfunctionisπ2(x)cΠ2x[1+O(ln(ln(x)))](1)(ln(x))2ln(x)whichisthebestthathasbeenproventhusfar.
Theconstantcin(1)hasbeenreducedto6.
8325,downfrompreviousvaluesashighas9[12].
TheformationofthisinequalityinvolvestwoofMerten'stheoremswhichwillbediscussedinthefollowingsection.
HardyandLittlewood[3]haveconjecturedthatc=2,andusingthisassumptionhaveformulatedwhatisnowcalledtheStrongTwinPrimeConjecture.
Inthefollowingexpression,abmeansthataapproaches1batthelimitsoftheexpressionsaandb.
Inthiscase,thelimitisasxapproachesinnity.
xdxπ2(x)2Π2(ln(x))2.
(2)2Anecessaryconditionforthestrongconjecture(2)isthattheprimegapsconstant,Δ≡limsupn→∞pn+1pnbeequaltozero.
ThemostrecentattemptedpnproofofthetwinprimeconjecturewasthatofArenstorf,in2004[1],butanerrorwasfoundshortlyafteritspublication,anditwaswithdrawn,leavingtheconjectureopentothisday.
2Mertens'TheoremsAnumberofimportantresultsaboutthespacingofprimenumberswerederivedbyFranzMertens,aGermanmathematicianofthelatenineteenthandearlytwentiethcentury.
ThefollowingproofsofMertens'conjecturesleaduptotheresultthatthesumofthereciprocalsofprimesdiverges,whichwillcontrast3TwinPrimeConjecturewithBrun'sconjecture,thatthesumofthereciprocalsoftwinprimesconverges.
First,weshouldbrieyshowthattheprimesareinnite,forotherwisetheimplicationsofMertens'theoremsarenotobvious.
Euclid'sproofofthispostulate,hissecondtheorem,isasfollows.
Let2,3,5,.
.
.
,pbeanenumerationofallprimenumbersuptop,andletq=(235·.
.
.
p)+1.
Thenqisnotdivisiblebyanyoftheprimesup···toandincludingp.
Therefore,itiseitherprimeordivisiblebyaprimebetweenpandq.
Intherstcase,qisaprimegreaterthanp.
Inthesecondcase,thedivisorofqbetweenpandqisaprimegreaterthanp.
Thenforanyprimep,thisconstructiongivesusaprimegreaterthanp.
Thus,thenumberofprimesmustbeinnite[4].
NowwecanresumewithMertens'theorems.
MertensTheorem1:Foranyrealnumberx≥1,x0≤ln(n)0suchthat11p=ln(ln(x))+b1+O(ln(x)),x≥2.
(6)p≤x6TwinPrimeConjectureProof:Wecanwrite1=ln(p)1=u(n)f(n)ppln(p)p≤xp≤xn≤xwhereu(n)=ln(pp)ifn=p,and0otherwise,andf(t)=ln(1t).
WedenenewfunctionsU(t)andg(t)asfollowsln(p)U(t)=u(n)==ln(t)+g(t)pn≤tp≤tThenU(t)=0fort3TheformulationoftheHardy-LittlewoodconjecturebuildsuponsomeofthetechniquesusedtoproveBrun'sconjecture,namelytheBrunsievetechniques.
TheBrunsievecanbeconstructedasfollows:LetXbeanonempty,nitesetofNobjects,andletP1,PrberdierentpropertiesthattheelementsofthesetXmighthave.
LetN0denotethenumberofelementsofXthathavenoneoftheseproperties.
ForanysubsetI={i1,ik}of{1,2,r},letN(1)=N(i1,ik)denotethenumberofelementsofXthathaveeachofthepropertiesPi1,Pi2Pik.
LetN()=|X|=N.
Ifmisanonnegativeeveninteger,thenmN0≤(1)kN(I).
(9)k=0|I|=kIfmisanonnegativeoddinteger,thenmN0≥(1)kN(I).
[8](10)k=0|I|=kTheproofgiveninNathanson[8]isasfollows.
LetxbeanelementofthesetX,andsupposethatxhasexactlylpropertiesPi.
Ifl=0,thenxiscountedonceinN0andonceinN(),butisnotcountedinN(I)ifIisnonempty.
Ifl≥1,thenxisnotcountedinN0.
Byrenumberingtheproperties,wecanassumethatxhasthepropertiesP1,P2,Pl.
LetI{1,2,l,r}.
Ifi∈Iforsomei>l,thenxisnotcountedinN(I).
IfI{1,2,l}thenxcontributes1toN(I).
Foreachk=0,1,l,thereareexactlyklsuchsubsetswith|I|=k.
Ifm≥l,thentheelementxcontributesll(1)k=0kk=0TwinPrimeConjecture9totherightsidesoftheinequalities.
Ifm2cln(ln(x)),then·rrcln(ln(x)))k1xy(·m≤x2k2cln(ln(x)).
Ifweletc=max{2c,(ln(2)1)},andlet·ln(y)1x=e(3c·ln(ln(y)))=y3c·ln(ln(y))m=2[cln(ln(y))]·Thensinceln(y)ln(x)=3c·ln(ln(y))yy(ln(ln(y)))22c·ln(ln(y))2,y4y4y4y2m<22c·ln(ln(y))=(ln(y))2c·ln(2)≤(ln(y))2Thenm2cln(ln(y))2c·ln(ln(y)ln(y))32x≤x·=exp(ln(ln(y)))=y3c·Finally,x(ln(ln(x)))2π2(x)<<.
(ln(x))2TwinPrimeConjecture126ConclusionThetwinprimeconjecturemayneverbeproven,butstudyingthepropertiesoftwinprimesiscertainlyarewardingexercise.
RecentworkonthetwinprimeconjecturebyDanGoldstonandCemYilidrimhasfocusedoncreatingexpressionsforthegapsizebetweenprimes,andinparticularfocusingontheexpressionΔ=liminfpn+1pn=1n→∞ln(pn)ResearchintobetterexpressionsfortheintervalbetweenconsecutiveprimesiscurrentlybeingconductedatStanford,sponsoredbytheAmericanInstituteofMathematics[12].
Thoughnumbertheoryhasbeenthefoundationofmanydierentbranchesofhighermathematics,itsfundamentalproblemsremaininterestingandfruitfulforresearchersinterestedinthepropertiesofprimenumbers.
References[1]Arenstorf,R.
F.
"ThereAreInnitelyManyPrimeTwins.
"26May2004.
http://arxiv.
org/abs/math.
NT/0405509.
[2]Guy,R.
K.
"GapsbetweenPrimes.
TwinPrimes.
"A8inUnsolvedProblemsinNumberTheory,2nded.
NewYork:Springer-Verlag,pp.
19-23,1994.
[3]Hardy,G.
H.
andLittlewood,J.
E.
"SomeProblemsof'PartitioNumerorum.
'III.
OntheExpressionofaNumberasaSumofPrimes.
"ActaMath.
44,1-70,1923.
[4]Hardy,G.
H.
andWright,E.
M.
AnIntroductiontotheTheoryofNumbers,5thed.
Oxford,England:ClarendonPress,1979.
[5]Havil,J.
Gamma:ExploringEuler'sConstant.
Princeton,NJ:PrincetonUniversityPress,pp.
30-31,2003.
[6]Miller,S.
J.
andTakloo-Bighash,R.
AnInvitationtoNumberTheory.
Princeton,NJ:PrincetonUniversityPress,pp.
326-328,2006.
[7]Narkiewicz,W.
TheDevelopmentofPrimeNumberTheory.
Berlin,Germany:SpringerPress,2000.
[8]Nathanson,M.
B.
AdditiveNumberTheory.
NewYork,NewYork:SpringerPress,1996.
[9]Ribenboim,P.
TheNewBookofPrimeNumberRecords.
NewYork:Springer-Verlag,pp.
261-265,1996.
[10]Shanks,D.
SolvedandUnsolvedProblemsinNumberTheory,4thed.
NewYork:Chelsea,p.
30,1993.
13TwinPrimeConjecture[11]Tenenbaum,G.
"ReArenstorf'spaperontheTwinPrimeConjecture.
"8Jun2004.
[12]Weisstein,EricW.
"TwinPrimeConjecture"http://mathworld.
wolfram.
com/TwinPrimeConjecture.
html,2006.
[13]Young,R.
M.
ExcursionsinCalculus.
TheMathematicalAssociationofAmerica,1992.
Therearemanyproofsfortheinnitudeofprimenumbers,butitisverydiculttoprovewhetherthereareaninnitenumberofpairsoftwinprimes.
Mostmathematiciansagreethattheevidencepointstowardthisconclusion,butnumerousattemptsataproofhavebeenfalsiedbysubsequentreview.
Theproblemitself,oneofthemostfamousopenproblemsinmathematics,hasyieldedanumberofrelatedresults,includingBrun'sconjecture,Mertens'theorems,andtheHardy-LittlewoodConjecture.
Alongwiththeseconjectures,thereareanumberofresultswhichareeasiertoarriveat,butneverthelesshelpmathematiciansthinkabouttheinnitudeofprimes,andthespecialpropertiesoftwinprimes.
Thispaperwillintroducetheaforementionedconjecturesassociatedwiththetwinprimeconjecture,andworkthroughsomeexercisesthatilluminatethedicultiesandintricaciesofthetwinprimeconjecture.
1Introduction:TheOriginalConjectureandFailedProofsThetermtwinprimewascoinedbyPaulStackelinthelatenineteenthcentury.
Sincethattime,mathematicianshavebeeninterestedinthepropertiesofrelatedprimes,bothinrelationtonumbertheoryasawhole,andasspecic,well-denedproblems.
Oneoftherstresultsoflookingattwinprimeswasthediscoverythat,asidefrom(3,5),alltwinprimesareoftheform6n±1.
Thiscomesfromnoticingthatanyprimegreaterthan3mustbeoftheform6n±1.
Toshowthis,notethatanyintegercanbewrittenas6x+y,wherexisanyinteger,andyis0,1,2,3,4or5.
Nowconsidereachyvalueindividually.
Wheny=0,6x+y=6xandisdivisibleby6.
Wheny=1therearenoimmediatelyrecognizablefactors,sothisisacandidateforprimacy.
Wheny=2,6x+2=2(3x+1),andsoisnotprime.
Forthecasewhen·y=3:6x+3=3(2x+1)andisnotprime.
Wheny=4:6x+4=2(3x+2)··andisnotprime.
Wheny=5,6x+5hasnoimmediatelyrecognizablefactors,andisthesecondcandidateforprimacy.
Thenallprimescanberepresentedaseither6n+1or6n1,andtwinprimes,sincetheyareseparatedbytwo,willhavetobe6n1and6n+1.
1TwinPrimeConjecture2Furtherresearchintotheconjecturehasbeenconcernedwithndingexpressionsforaformoftheprimecountingfunctionπ(x)thatdependonthetwinprimeconstant.
Theprimecountingfunctionisdenedasπ(x)={N(p)|px}whereN(p)denotesthenumberofprimes,p.
Onemotivationfordeningtheprimecountingfunctionisthatitcanbeusedtodetermineaformulaforthesizeoftheintervalsbetweenprimes,aswellasgivingusanindicationoftherateofdecaybywhichprimesthinoutinhighernumbers.
Ithasbeenshownalgebraicallythattheprimecountingfunctionincreasesasymptoticallywiththelogarithmicintegral[12].
Inthefollowingexpression,π2(x)referstothenumberofprimesoftheformpandp+2greaterthanx,andisthetwin2primeconstant,whichisdenedbytheexpression(19p11)2)overprimesp2.
ThetermO(x),meaning"ontheorderofx,"isdenedasfollows:iff(x)andg(x)aretwofunctionsdenedonthesameset,thenf(x)isO(g(x))asxgoestoinnityifandonlyifthereexistssomex0andsomeMsuchthat|f(x)|M|g(x)|forxgreaterthanx0.
Thisexpressionforthetwinprimecountingfunctionisπ2(x)cΠ2x[1+O(ln(ln(x)))](1)(ln(x))2ln(x)whichisthebestthathasbeenproventhusfar.
Theconstantcin(1)hasbeenreducedto6.
8325,downfrompreviousvaluesashighas9[12].
TheformationofthisinequalityinvolvestwoofMerten'stheoremswhichwillbediscussedinthefollowingsection.
HardyandLittlewood[3]haveconjecturedthatc=2,andusingthisassumptionhaveformulatedwhatisnowcalledtheStrongTwinPrimeConjecture.
Inthefollowingexpression,abmeansthataapproaches1batthelimitsoftheexpressionsaandb.
Inthiscase,thelimitisasxapproachesinnity.
xdxπ2(x)2Π2(ln(x))2.
(2)2Anecessaryconditionforthestrongconjecture(2)isthattheprimegapsconstant,Δ≡limsupn→∞pn+1pnbeequaltozero.
ThemostrecentattemptedpnproofofthetwinprimeconjecturewasthatofArenstorf,in2004[1],butanerrorwasfoundshortlyafteritspublication,anditwaswithdrawn,leavingtheconjectureopentothisday.
2Mertens'TheoremsAnumberofimportantresultsaboutthespacingofprimenumberswerederivedbyFranzMertens,aGermanmathematicianofthelatenineteenthandearlytwentiethcentury.
ThefollowingproofsofMertens'conjecturesleaduptotheresultthatthesumofthereciprocalsofprimesdiverges,whichwillcontrast3TwinPrimeConjecturewithBrun'sconjecture,thatthesumofthereciprocalsoftwinprimesconverges.
First,weshouldbrieyshowthattheprimesareinnite,forotherwisetheimplicationsofMertens'theoremsarenotobvious.
Euclid'sproofofthispostulate,hissecondtheorem,isasfollows.
Let2,3,5,.
.
.
,pbeanenumerationofallprimenumbersuptop,andletq=(235·.
.
.
p)+1.
Thenqisnotdivisiblebyanyoftheprimesup···toandincludingp.
Therefore,itiseitherprimeordivisiblebyaprimebetweenpandq.
Intherstcase,qisaprimegreaterthanp.
Inthesecondcase,thedivisorofqbetweenpandqisaprimegreaterthanp.
Thenforanyprimep,thisconstructiongivesusaprimegreaterthanp.
Thus,thenumberofprimesmustbeinnite[4].
NowwecanresumewithMertens'theorems.
MertensTheorem1:Foranyrealnumberx≥1,x0≤ln(n)0suchthat11p=ln(ln(x))+b1+O(ln(x)),x≥2.
(6)p≤x6TwinPrimeConjectureProof:Wecanwrite1=ln(p)1=u(n)f(n)ppln(p)p≤xp≤xn≤xwhereu(n)=ln(pp)ifn=p,and0otherwise,andf(t)=ln(1t).
WedenenewfunctionsU(t)andg(t)asfollowsln(p)U(t)=u(n)==ln(t)+g(t)pn≤tp≤tThenU(t)=0fort3TheformulationoftheHardy-LittlewoodconjecturebuildsuponsomeofthetechniquesusedtoproveBrun'sconjecture,namelytheBrunsievetechniques.
TheBrunsievecanbeconstructedasfollows:LetXbeanonempty,nitesetofNobjects,andletP1,PrberdierentpropertiesthattheelementsofthesetXmighthave.
LetN0denotethenumberofelementsofXthathavenoneoftheseproperties.
ForanysubsetI={i1,ik}of{1,2,r},letN(1)=N(i1,ik)denotethenumberofelementsofXthathaveeachofthepropertiesPi1,Pi2Pik.
LetN()=|X|=N.
Ifmisanonnegativeeveninteger,thenmN0≤(1)kN(I).
(9)k=0|I|=kIfmisanonnegativeoddinteger,thenmN0≥(1)kN(I).
[8](10)k=0|I|=kTheproofgiveninNathanson[8]isasfollows.
LetxbeanelementofthesetX,andsupposethatxhasexactlylpropertiesPi.
Ifl=0,thenxiscountedonceinN0andonceinN(),butisnotcountedinN(I)ifIisnonempty.
Ifl≥1,thenxisnotcountedinN0.
Byrenumberingtheproperties,wecanassumethatxhasthepropertiesP1,P2,Pl.
LetI{1,2,l,r}.
Ifi∈Iforsomei>l,thenxisnotcountedinN(I).
IfI{1,2,l}thenxcontributes1toN(I).
Foreachk=0,1,l,thereareexactlyklsuchsubsetswith|I|=k.
Ifm≥l,thentheelementxcontributesll(1)k=0kk=0TwinPrimeConjecture9totherightsidesoftheinequalities.
Ifm2cln(ln(x)),then·rrcln(ln(x)))k1xy(·m≤x2k2cln(ln(x)).
Ifweletc=max{2c,(ln(2)1)},andlet·ln(y)1x=e(3c·ln(ln(y)))=y3c·ln(ln(y))m=2[cln(ln(y))]·Thensinceln(y)ln(x)=3c·ln(ln(y))yy(ln(ln(y)))22c·ln(ln(y))2,y4y4y4y2m<22c·ln(ln(y))=(ln(y))2c·ln(2)≤(ln(y))2Thenm2cln(ln(y))2c·ln(ln(y)ln(y))32x≤x·=exp(ln(ln(y)))=y3c·Finally,x(ln(ln(x)))2π2(x)<<.
(ln(x))2TwinPrimeConjecture126ConclusionThetwinprimeconjecturemayneverbeproven,butstudyingthepropertiesoftwinprimesiscertainlyarewardingexercise.
RecentworkonthetwinprimeconjecturebyDanGoldstonandCemYilidrimhasfocusedoncreatingexpressionsforthegapsizebetweenprimes,andinparticularfocusingontheexpressionΔ=liminfpn+1pn=1n→∞ln(pn)ResearchintobetterexpressionsfortheintervalbetweenconsecutiveprimesiscurrentlybeingconductedatStanford,sponsoredbytheAmericanInstituteofMathematics[12].
Thoughnumbertheoryhasbeenthefoundationofmanydierentbranchesofhighermathematics,itsfundamentalproblemsremaininterestingandfruitfulforresearchersinterestedinthepropertiesofprimenumbers.
References[1]Arenstorf,R.
F.
"ThereAreInnitelyManyPrimeTwins.
"26May2004.
http://arxiv.
org/abs/math.
NT/0405509.
[2]Guy,R.
K.
"GapsbetweenPrimes.
TwinPrimes.
"A8inUnsolvedProblemsinNumberTheory,2nded.
NewYork:Springer-Verlag,pp.
19-23,1994.
[3]Hardy,G.
H.
andLittlewood,J.
E.
"SomeProblemsof'PartitioNumerorum.
'III.
OntheExpressionofaNumberasaSumofPrimes.
"ActaMath.
44,1-70,1923.
[4]Hardy,G.
H.
andWright,E.
M.
AnIntroductiontotheTheoryofNumbers,5thed.
Oxford,England:ClarendonPress,1979.
[5]Havil,J.
Gamma:ExploringEuler'sConstant.
Princeton,NJ:PrincetonUniversityPress,pp.
30-31,2003.
[6]Miller,S.
J.
andTakloo-Bighash,R.
AnInvitationtoNumberTheory.
Princeton,NJ:PrincetonUniversityPress,pp.
326-328,2006.
[7]Narkiewicz,W.
TheDevelopmentofPrimeNumberTheory.
Berlin,Germany:SpringerPress,2000.
[8]Nathanson,M.
B.
AdditiveNumberTheory.
NewYork,NewYork:SpringerPress,1996.
[9]Ribenboim,P.
TheNewBookofPrimeNumberRecords.
NewYork:Springer-Verlag,pp.
261-265,1996.
[10]Shanks,D.
SolvedandUnsolvedProblemsinNumberTheory,4thed.
NewYork:Chelsea,p.
30,1993.
13TwinPrimeConjecture[11]Tenenbaum,G.
"ReArenstorf'spaperontheTwinPrimeConjecture.
"8Jun2004.
[12]Weisstein,EricW.
"TwinPrimeConjecture"http://mathworld.
wolfram.
com/TwinPrimeConjecture.
html,2006.
[13]Young,R.
M.
ExcursionsinCalculus.
TheMathematicalAssociationofAmerica,1992.
- decaylet美人双胞胎姐妹相关文档
- 师兄let美人双胞胎姐妹
- 乌尔let美人双胞胎姐妹
- 江西省第三届"艺德杯"中小学绘画书法作品
- 科研let美人双胞胎姐妹
- 正旦let美人双胞胎姐妹
- 电影let美人双胞胎姐妹
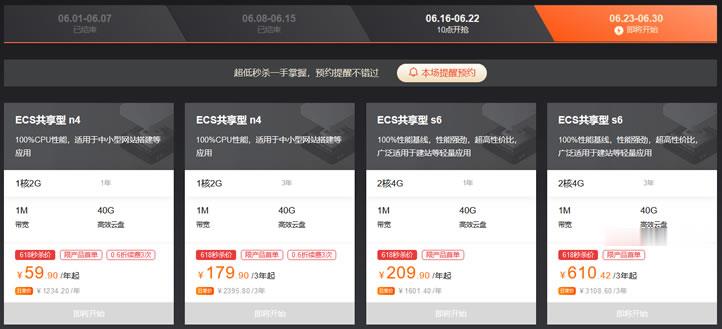
阿里云年中活动最后一周 - ECS共享型N4 2G1M年付59元
以前我们在参与到云服务商促销活动的时候周期基本是一周时间,而如今我们会看到无论是云服务商还是电商活动基本上周期都要有超过一个月,所以我们有一些网友习惯在活动结束之前看看商家是不是有最后的促销活动吸引力的,比如有看到阿里云年中活动最后一周,如果我们有需要云服务器的可以看看。在前面的文章中(阿里云新人福利选择共享性N4云服务器年79.86元且送2月数据库),(LAOZUO.ORG)有提到阿里云今年的云...
限时新网有提供5+个免费域名
有在六月份的时候也有分享过新网域名注册商发布的域名促销活动(这里)。这不在九月份发布秋季域名促销活动,有提供年付16元的.COM域名,同时还有5个+的特殊后缀的域名是免费的。对于新网服务商是曾经非常老牌的域名注册商,早年也是有在他们家注册域名的。我们可以看到,如果有针对新用户的可以领到16元的.COM域名。包括还有首年免费的.XYZ、.SHOP、Space等等后缀的域名。除了.COM域名之外的其他...

georgedatacenter:美国VPS可选洛杉矶/芝加哥/纽约/达拉斯机房,$20/年;洛杉矶独立服务器39美元/月
georgedatacenter怎么样?georgedatacenter这次其实是两个促销,一是促销一款特价洛杉矶E3-1220 V5独服,性价比其实最高;另外还促销三款特价vps,大家可以根据自己的需要入手。georgedatacenter是一家成立于2019年的美国vps商家,主营美国洛杉矶、芝加哥、达拉斯、新泽西、西雅图机房的VPS、邮件服务器和托管独立服务器业务。georgedatacen...
let美人双胞胎姐妹为你推荐
-
.net虚拟主机虚拟主机 net framework 1.1/2.0/3.5 什么意思?域名空间代理我想做域名空间代理!免费网站空间免费网站空间哪个好国内最好的虚拟主机国内虚拟主机哪家的好?大连虚拟主机大连哪些地方的网通机房好?淘宝虚拟主机请问在淘宝的代购国外虚拟主机可以买吗?长沙虚拟主机长沙哪里虚拟主机和主机托管比较关好!四川虚拟主机四川java虚拟主机,哪里比较好双线虚拟主机什么是智能双线虚拟主机?联动天下的双线主机有什么优势?中文域名中文域名是怎么回事?